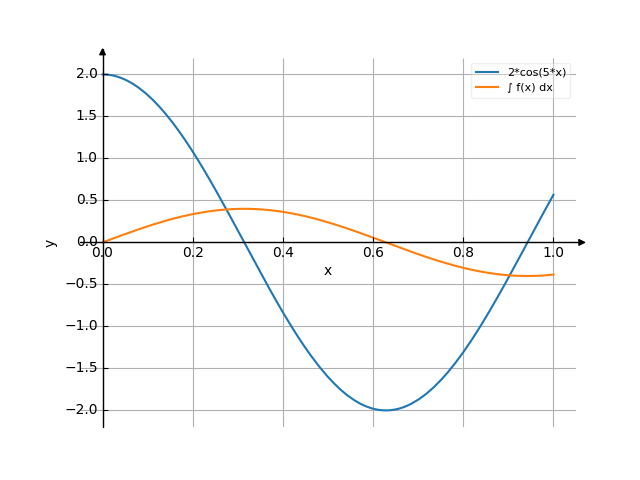
Integral of 2cos5x dx

You have entered [src]

  1              
  /              
 |               
 |  2*cos(5*x) dx
 |               
/                
0

\int\limits_{0}^{1} 2 \cos{\left(5 x \right)}\, dx

Simplify

Detail solution

The integral of a constant times a function is the constant times the integral of the function:

$\int 2 \cos{\left(5 x \right)}\, dx = 2 \int \cos{\left(5 x \right)}\, dx$
1. Let $u = 5 x$ .
  
  Then let $du = 5 dx$ and substitute $\frac{du}{5}$ :
  
  $\int \frac{\cos{\left(u \right)}}{25}\, du$
  1. The integral of a constant times a function is the constant times the integral of the function:
    
    $\int \frac{\cos{\left(u \right)}}{5}\, du = \frac{\int \cos{\left(u \right)}\, du}{5}$
    1. The integral of cosine is sine:
      
      $\int \cos{\left(u \right)}\, du = \sin{\left(u \right)}$
    So, the result is: $\frac{\sin{\left(u \right)}}{5}$
  Now substitute $u$ back in:
  
  $\frac{\sin{\left(5 x \right)}}{5}$
So, the result is: $\frac{2 \sin{\left(5 x \right)}}{5}$
Add the constant of integration:

$\frac{2 \sin{\left(5 x \right)}}{5}+ \mathrm{constant}$

The answer is:

\frac{2 \sin{\left(5 x \right)}}{5}+ \mathrm{constant}

The answer (Indefinite) [src]

  /                              
 |                     2*sin(5*x)
 | 2*cos(5*x) dx = C + ----------
 |                         5     
/

{{2\,\sin \left(5\,x\right)}\over{5}}

Simplify

The graph

Plot the graph f(x)

The answer [src]

2*sin(5)
--------
   5

{{2\,\sin 5}\over{5}}

2*sin(5)
--------
   5

\frac{2 \sin{\left(5 \right)}}{5}

Simplify

Numerical answer [src]

-0.383569709865255

-0.383569709865255

The graph

Use the examples entering the upper and lower limits of integration.