Mister Exam
Other calculators
- Taylor Series Step by Step
- Fourier series step by step
- Differential equations Step by Step
- Product of series
-
How to use it?
- Sum of series:
-
(7^n-2^n)/14^n
-
3^(n-1)/8^(n-1)
-
(n^2)(tg(pi/2)^5)
- (3*k-5)*(3*k+6)-(3*k-11)*(3*k+12)
-
Identical expressions
- one /(eight +(x+ two)n)
- 1 divide by (8 plus (x plus 2)n)
- one divide by (eight plus (x plus two)n)
- 1/8+x+2n
- 1 divide by (8+(x+2)n)
-
Similar expressions
- 1/(8-(x+2)n)
- 1/(8+(x-2)n)
Sum of series 1/(8+(x+2)n)
The solution
You have entered
[src]
oo ___ \ ` \ 1 ) ------------- / 8 + (x + 2)*n /__, n = 1
$$\sum_{n=1}^{\infty} \frac{1}{n \left(x + 2\right) + 8}$$
Sum(1/(8 + (x + 2)*n), (n, 1, oo))
The radius of convergence of the power series
Given number:
$$\frac{1}{n \left(x + 2\right) + 8}$$
It is a series of species
$$a_{n} \left(c x - x_{0}\right)^{d n}$$
- power series.
The radius of convergence of a power series can be calculated by the formula:
$$R^{d} = \frac{x_{0} + \lim_{n \to \infty} \left|{\frac{a_{n}}{a_{n + 1}}}\right|}{c}$$
In this case
$$a_{n} = \frac{1}{n \left(x + 2\right) + 8}$$
and
$$x_{0} = 0$$
,
$$d = 0$$
,
$$c = 1$$
then
$$1 = \lim_{n \to \infty} \left|{\frac{\left(n + 1\right) \left(x + 2\right) + 8}{n \left(x + 2\right) + 8}}\right|$$
Let's take the limit
we find
$$\frac{1}{n \left(x + 2\right) + 8}$$
It is a series of species
$$a_{n} \left(c x - x_{0}\right)^{d n}$$
- power series.
The radius of convergence of a power series can be calculated by the formula:
$$R^{d} = \frac{x_{0} + \lim_{n \to \infty} \left|{\frac{a_{n}}{a_{n + 1}}}\right|}{c}$$
In this case
$$a_{n} = \frac{1}{n \left(x + 2\right) + 8}$$
and
$$x_{0} = 0$$
,
$$d = 0$$
,
$$c = 1$$
then
$$1 = \lim_{n \to \infty} \left|{\frac{\left(n + 1\right) \left(x + 2\right) + 8}{n \left(x + 2\right) + 8}}\right|$$
Let's take the limit
we find
True
False
The answer
[src]
/ / 12 2*x \ / 2 x \ | Gamma|----- + -----|*Gamma|-1 + ----- + -----| 2 | \2 + x 2 + x/ \ 2 + x 2 + x/ im (x) (2 + re(x))*(10 + re(x)) (2 + re(x))*(12 + 2*re(x)) |------------------------------------------------------- for 1 - --------------------- + ------------------------ - -------------------------- < 0 | / 2 x \ / 12 2*x \ 2 2 2 2 2 2 |(10 + x)*Gamma|----- + -----|*Gamma|-1 + ----- + -----| (2 + re(x)) + im (x) (2 + re(x)) + im (x) (2 + re(x)) + im (x) | \2 + x 2 + x/ \ 2 + x 2 + x/ | < oo | ___ | \ ` | \ 1 | ) ------------- otherwise | / 8 + 2*n + n*x | /__, | n = 1 \
$$\begin{cases} \frac{\Gamma\left(\frac{2 x}{x + 2} + \frac{12}{x + 2}\right) \Gamma\left(\frac{x}{x + 2} - 1 + \frac{2}{x + 2}\right)}{\left(x + 10\right) \Gamma\left(\frac{x}{x + 2} + \frac{2}{x + 2}\right) \Gamma\left(\frac{2 x}{x + 2} - 1 + \frac{12}{x + 2}\right)} & \text{for}\: 1 + \frac{\left(\operatorname{re}{\left(x\right)} + 2\right) \left(\operatorname{re}{\left(x\right)} + 10\right)}{\left(\operatorname{re}{\left(x\right)} + 2\right)^{2} + \left(\operatorname{im}{\left(x\right)}\right)^{2}} - \frac{\left(\operatorname{re}{\left(x\right)} + 2\right) \left(2 \operatorname{re}{\left(x\right)} + 12\right)}{\left(\operatorname{re}{\left(x\right)} + 2\right)^{2} + \left(\operatorname{im}{\left(x\right)}\right)^{2}} - \frac{\left(\operatorname{im}{\left(x\right)}\right)^{2}}{\left(\operatorname{re}{\left(x\right)} + 2\right)^{2} + \left(\operatorname{im}{\left(x\right)}\right)^{2}} < 0 \\\sum_{n=1}^{\infty} \frac{1}{n x + 2 n + 8} & \text{otherwise} \end{cases}$$
Piecewise((gamma(12/(2 + x) + 2*x/(2 + x))*gamma(-1 + 2/(2 + x) + x/(2 + x))/((10 + x)*gamma(2/(2 + x) + x/(2 + x))*gamma(-1 + 12/(2 + x) + 2*x/(2 + x))), 1 - im(x)^2/((2 + re(x))^2 + im(x)^2) + (2 + re(x))*(10 + re(x))/((2 + re(x))^2 + im(x)^2) - (2 + re(x))*(12 + 2*re(x))/((2 + re(x))^2 + im(x)^2) < 0), (Sum(1/(8 + 2*n + n*x), (n, 1, oo)), True))
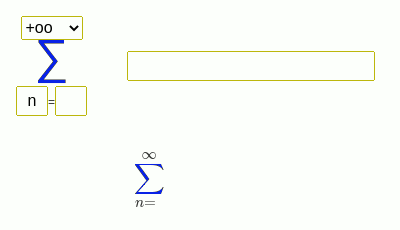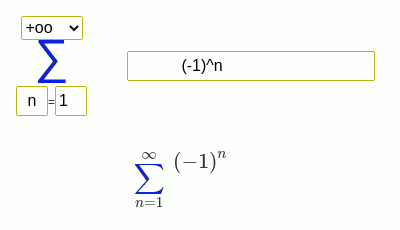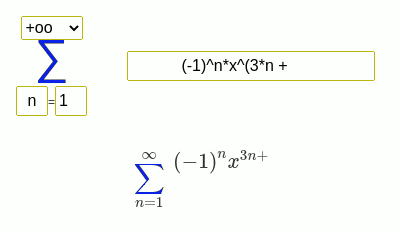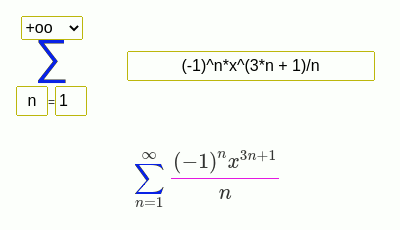
Examples of finding the sum of a series
- The Sum of the Power Series
x^n/n
(x-1)^n
- Factorial
1/2^(n!)
n^2/n!
x^n/n!
k!/(n!*(n+k)!)
- Flint Hills Series
csc(n)^2/n^3
- Basel problem
1/n^2
1/n^4
1/n^6
- Harmonic series
1/n
- Grandi's series
(-1)^n
- Alternating series
(-1)^(n + 1)/n
(n + 2)*(-1)^(n - 1)
(3*n - 1)/(-5)^n
(-1)^(n - 1)*n/(6*n - 5)
- Newton–Mercator series
(-1)^(n + 1)/n*x^n
- Exam the series for convergence
(3*n - 1)/(-5)^n