Mister Exam

Lang:

Other calculators

How to use it?
Integral of d{x}:
Integral of sin(5x)cos(2x)dx
Integral of 3*sinx*cosx
Integral of 1/(4-3cos(x))
Integral of dx/sqrt(4-25x^2)
Identical expressions
dx/sqrt(four - two 5x^2)
dx divide by square root of (4 minus 25x squared )
dx divide by square root of (four minus two 5x squared )
dx/√(4-25x^2)
dx/sqrt(4-25x2)
dx/sqrt4-25x2
dx/sqrt(4-25x²)
dx/sqrt(4-25x to the power of 2)
dx/sqrt4-25x^2
dx divide by sqrt(4-25x^2)
Similar expressions
dx/sqrt(4+25x^2)

Solving integrals/ dx/sqrt(4-25x^2)

Integral of dx/sqrt(4-25x^2) dx

The solution

You have entered [src]

  1                    
  /                    
 |                     
 |          1          
 |  1*-------------- dx
 |       ___________   
 |      /         2    
 |    \/  4 - 25*x     
 |                     
/                      
0

$$\int\limits_{0}^{1} 1 \cdot \frac{1}{\sqrt{- 25 x^{2} + 4}}\, dx$$

Simplify

Detail solution

TrigSubstitutionRule(theta=_theta, func=2*sin(_theta)/5, rewritten=1/5, substep=ConstantRule(constant=1/5, context=1/5, symbol=_theta), restriction=(x > -2/5) & (x < 2/5), context=1/sqrt(4 - 25*x**2), symbol=x)

Now simplify:

$\begin{cases} \frac{\operatorname{asin}{\left(\frac{5 x}{2} \right)}}{5} & \text{for}\: x > - \frac{2}{5} \wedge x < \frac{2}{5} \\\text{NaN} & \text{otherwise} \end{cases}$
Add the constant of integration:

$\begin{cases} \frac{\operatorname{asin}{\left(\frac{5 x}{2} \right)}}{5} & \text{for}\: x > - \frac{2}{5} \wedge x < \frac{2}{5} \\\text{NaN} & \text{otherwise} \end{cases}+ \mathrm{constant}$

The answer is:

$\begin{cases} \frac{\operatorname{asin}{\left(\frac{5 x}{2} \right)}}{5} & \text{for}\: x > - \frac{2}{5} \wedge x < \frac{2}{5} \\\text{NaN} & \text{otherwise} \end{cases}+ \mathrm{constant}$

The answer (Indefinite) [src]

  /                                                                  
 |                           //    /5*x\                            \
 |         1                 ||asin|---|                            |
 | 1*-------------- dx = C + |<    \ 2 /                            |
 |      ___________          ||---------  for And(x > -2/5, x < 2/5)|
 |     /         2           \\    5                                /
 |   \/  4 - 25*x                                                    
 |                                                                   
/

$${{\arcsin \left({{5\,x}\over{2}}\right)}\over{5}}$$

Simplify

The graph

Plot the graph f(x)

The answer [src]

asin(5/2)
---------
    5

$${{\arcsin \left({{5}\over{2}}\right)}\over{5}}$$

asin(5/2)
---------
    5

$$\frac{\operatorname{asin}{\left(\frac{5}{2} \right)}}{5}$$

Simplify

Numerical answer [src]

(0.286954509007177 - 0.320835134585762j)

(0.286954509007177 - 0.320835134585762j)

The graph

Use the examples entering the upper and lower limits of integration.

Other calculators

How to use it?

Identical expressions

Similar expressions

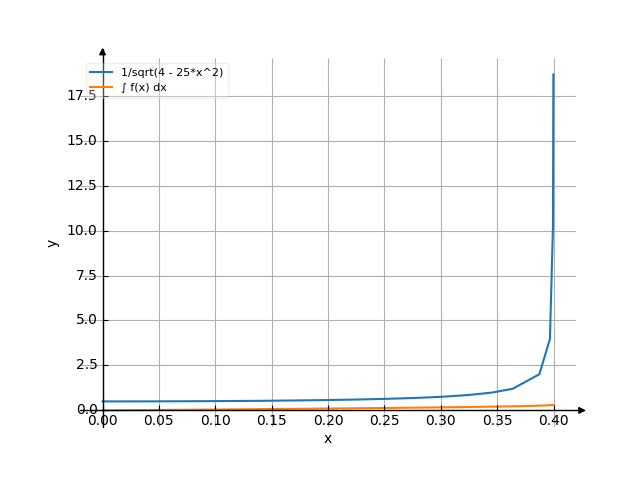
Integral of dx/sqrt(4-25x^2) dx

Limits of integration:

The graph:

Piecewise:

The solution