log1:7(7𝑥−3)=log1(5𝑥+11) equation
The teacher will be very surprised to see your correct solution 😉
The solution
You have entered
[src]
log(1) log(5*x + 11) ------*(7*x - 3) = ------------- 7 log(1)
$$\frac{\log{\left(1 \right)}}{7} \left(7 x - 3\right) = \frac{\log{\left(5 x + 11 \right)}}{\log{\left(1 \right)}}$$
Detail solution
Given the equation
$$\frac{\log{\left(1 \right)}}{7} \left(7 x - 3\right) = \frac{\log{\left(5 x + 11 \right)}}{\log{\left(1 \right)}}$$
Transfer the right side of the equation left part with negative sign
$$\tilde{\infty} \log{\left(5 x + 11 \right)} = 0$$
Let's divide both parts of the equation by the multiplier of log =±oo
$$\log{\left(5 x + 11 \right)} = 0$$
This equation is of the form:
By definition log
then
$$5 x + 11 = e^{\frac{0}{\tilde{\infty}}}$$
simplify
$$5 x + 11 = 1$$
$$5 x = -10$$
$$x = -2$$
$$\frac{\log{\left(1 \right)}}{7} \left(7 x - 3\right) = \frac{\log{\left(5 x + 11 \right)}}{\log{\left(1 \right)}}$$
Transfer the right side of the equation left part with negative sign
$$\tilde{\infty} \log{\left(5 x + 11 \right)} = 0$$
Let's divide both parts of the equation by the multiplier of log =±oo
$$\log{\left(5 x + 11 \right)} = 0$$
This equation is of the form:
log(v)=p
By definition log
v=e^p
then
$$5 x + 11 = e^{\frac{0}{\tilde{\infty}}}$$
simplify
$$5 x + 11 = 1$$
$$5 x = -10$$
$$x = -2$$

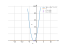 Equation 16x+5x^2+12=0
Equation 16x+5x^2+12=0
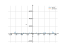 Equation tan(x)=sqrt(3)/3
Equation tan(x)=sqrt(3)/3