Mister Exam

Other calculators

y=tgsqrtxarcctg3x^5
The derivative of the function/ y=tgsqrtxarcctg3x^5

Derivative of y=tgsqrtxarcctg3x^5

Function f() - derivative -N order at the point
v

The graph:

from to

Piecewise:

The solution

You have entered [src] 
   /  ___\     5     
tan\\/ x /*acot (3*x)
tan⁡(x)acot⁡5(3x)\tan{\left(\sqrt{x} \right)} \operatorname{acot}^{5}{\left(3 x \right)} 
d /   /  ___\     5     \
--\tan\\/ x /*acot (3*x)/
dx
ddxtan⁡(x)acot⁡5(3x)\frac{d}{d x} \tan{\left(\sqrt{x} \right)} \operatorname{acot}^{5}{\left(3 x \right)}
Transform
The graph
02468-8-6-4-2-1010-1010
Plot the graph f(x)
Plot the graph f'(x)
The first derivative [src] 
    5      /       2/  ___\\          4         /  ___\
acot (3*x)*\1 + tan \\/ x //   15*acot (3*x)*tan\\/ x /
---------------------------- - ------------------------
              ___                             2        
          2*\/ x                       1 + 9*x
−15tan⁡(x)acot⁡4(3x)9x2+1+(tan⁡2(x)+1)acot⁡5(3x)2x- \frac{15 \tan{\left(\sqrt{x} \right)} \operatorname{acot}^{4}{\left(3 x \right)}}{9 x^{2} + 1} + \frac{\left(\tan^{2}{\left(\sqrt{x} \right)} + 1\right) \operatorname{acot}^{5}{\left(3 x \right)}}{2 \sqrt{x}}
Simplify
The second derivative [src] 
           /                                                                 /              /  ___\\                                 \
           |                                        2      /       2/  ___\\ |   1     2*tan\\/ x /|                                 |
           |                                    acot (3*x)*\1 + tan \\/ x //*|- ---- + ------------|                                 |
           |                          /  ___\                                |   3/2        x      |      /       2/  ___\\          |
    3      |90*(2 + 3*x*acot(3*x))*tan\\/ x /                                \  x                  /   15*\1 + tan \\/ x //*acot(3*x)|
acot (3*x)*|--------------------------------- + ---------------------------------------------------- - ------------------------------|
           |                     2                                       4                                      ___ /       2\       |
           |           /       2\                                                                             \/ x *\1 + 9*x /       |
           \           \1 + 9*x /                                                                                                    /
((2tan⁡(x)x−1x32)(tan⁡2(x)+1)acot⁡2(3x)4+90⋅(3xacot⁡(3x)+2)tan⁡(x)(9x2+1)2−15(tan⁡2(x)+1)acot⁡(3x)x(9x2+1))acot⁡3(3x)\left(\frac{\left(\frac{2 \tan{\left(\sqrt{x} \right)}}{x} - \frac{1}{x^{\frac{3}{2}}}\right) \left(\tan^{2}{\left(\sqrt{x} \right)} + 1\right) \operatorname{acot}^{2}{\left(3 x \right)}}{4} + \frac{90 \cdot \left(3 x \operatorname{acot}{\left(3 x \right)} + 2\right) \tan{\left(\sqrt{x} \right)}}{\left(9 x^{2} + 1\right)^{2}} - \frac{15 \left(\tan^{2}{\left(\sqrt{x} \right)} + 1\right) \operatorname{acot}{\left(3 x \right)}}{\sqrt{x} \left(9 x^{2} + 1\right)}\right) \operatorname{acot}^{3}{\left(3 x \right)}
Simplify
The third derivative [src] 
           /      /                                               2     2     \                                           /            /  ___\     /       2/  ___\\        2/  ___\\                                   /              /  ___\\                                                      \
           |      |      2           6       36*x*acot(3*x)   36*x *acot (3*x)|    /  ___\       3      /       2/  ___\\ | 3     6*tan\\/ x /   2*\1 + tan \\/ x //   4*tan \\/ x /|          2      /       2/  ___\\ |   1     2*tan\\/ x /|                                                      |
           |  270*|- acot (3*x) + -------- + -------------- + ----------------|*tan\\/ x /   acot (3*x)*\1 + tan \\/ x //*|---- - ------------ + ------------------- + -------------|   45*acot (3*x)*\1 + tan \\/ x //*|- ---- + ------------|                                                      |
           |      |                      2             2                 2    |                                           | 5/2         2                 3/2                3/2    |                                   |   3/2        x      |       /       2/  ___\\                              |
    2      |      \               1 + 9*x       1 + 9*x           1 + 9*x     /                                           \x           x                 x                  x       /                                   \  x                  /   135*\1 + tan \\/ x //*(2 + 3*x*acot(3*x))*acot(3*x)|
acot (3*x)*|- ---------------------------------------------------------------------------- + ---------------------------------------------------------------------------------------- - ------------------------------------------------------- + ---------------------------------------------------|
           |                                            2                                                                               8                                                                       /       2\                                                         2                 |
           |                                  /       2\                                                                                                                                                      4*\1 + 9*x /                                           ___ /       2\                  |
           \                                  \1 + 9*x /                                                                                                                                                                                                           \/ x *\1 + 9*x /                  /
((tan⁡2(x)+1)(−6tan⁡(x)x2+2(tan⁡2(x)+1)x32+4tan⁡2(x)x32+3x52)acot⁡3(3x)8−45⋅(2tan⁡(x)x−1x32)(tan⁡2(x)+1)acot⁡2(3x)4⋅(9x2+1)−270⋅(36x2acot⁡2(3x)9x2+1+36xacot⁡(3x)9x2+1−acot⁡2(3x)+69x2+1)tan⁡(x)(9x2+1)2+135⋅(3xacot⁡(3x)+2)(tan⁡2(x)+1)acot⁡(3x)x(9x2+1)2)acot⁡2(3x)\left(\frac{\left(\tan^{2}{\left(\sqrt{x} \right)} + 1\right) \left(- \frac{6 \tan{\left(\sqrt{x} \right)}}{x^{2}} + \frac{2 \left(\tan^{2}{\left(\sqrt{x} \right)} + 1\right)}{x^{\frac{3}{2}}} + \frac{4 \tan^{2}{\left(\sqrt{x} \right)}}{x^{\frac{3}{2}}} + \frac{3}{x^{\frac{5}{2}}}\right) \operatorname{acot}^{3}{\left(3 x \right)}}{8} - \frac{45 \cdot \left(\frac{2 \tan{\left(\sqrt{x} \right)}}{x} - \frac{1}{x^{\frac{3}{2}}}\right) \left(\tan^{2}{\left(\sqrt{x} \right)} + 1\right) \operatorname{acot}^{2}{\left(3 x \right)}}{4 \cdot \left(9 x^{2} + 1\right)} - \frac{270 \cdot \left(\frac{36 x^{2} \operatorname{acot}^{2}{\left(3 x \right)}}{9 x^{2} + 1} + \frac{36 x \operatorname{acot}{\left(3 x \right)}}{9 x^{2} + 1} - \operatorname{acot}^{2}{\left(3 x \right)} + \frac{6}{9 x^{2} + 1}\right) \tan{\left(\sqrt{x} \right)}}{\left(9 x^{2} + 1\right)^{2}} + \frac{135 \cdot \left(3 x \operatorname{acot}{\left(3 x \right)} + 2\right) \left(\tan^{2}{\left(\sqrt{x} \right)} + 1\right) \operatorname{acot}{\left(3 x \right)}}{\sqrt{x} \left(9 x^{2} + 1\right)^{2}}\right) \operatorname{acot}^{2}{\left(3 x \right)}
Simplify
The graph
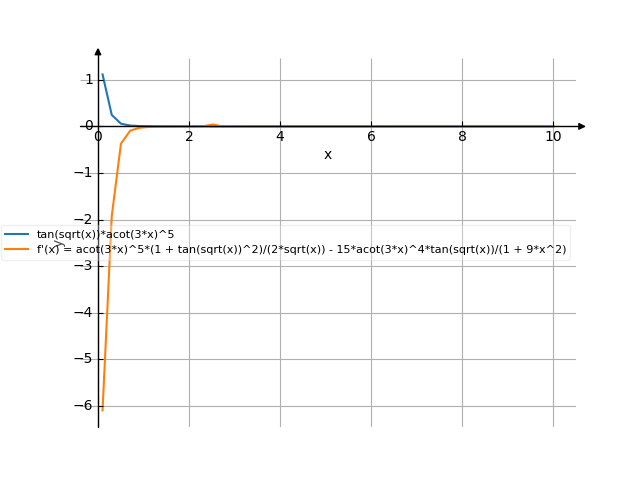
Derivative of y=tgsqrtxarcctg3x^5
    © Mister Exam – Calculator