Mister Exam
Other calculators
- Derivative of a implicit defined function
- Derivative of Parametric Function
- Partial derivative of the function
- Curve tracing functions Step by Step
- Integral Step by Step
- Differential equations Step by Step
- Limits Step by Step
-
How to use it?
- Derivative of:
-
Derivative of sin²2x
-
Derivative of sec^3x
-
Derivative of (3x+5)^6
-
Derivative of y'=sqrt(x)*ln(x)
- Integral of d{x}:
-
sin²2x
-
Identical expressions
- sin²2x
- sinus of ²2x
-
Similar expressions
- ((1\4)sin²(2x))
- y=sin²(2x+3)
- f(x)=xsin²(2x)
Derivative of sin²2x
The solution
You have entered
[src]
22 sin (x)
$$\sin^{22}{\left(x \right)}$$
d / 22 \ --\sin (x)/ dx
$$\frac{d}{d x} \sin^{22}{\left(x \right)}$$
Detail solution
-
Let .
-
Apply the power rule: goes to
-
Then, apply the chain rule. Multiply by :
-
The derivative of sine is cosine:
The result of the chain rule is:
-
The answer is:
The first derivative
[src]
21 22*sin (x)*cos(x)
$$22 \sin^{21}{\left(x \right)} \cos{\left(x \right)}$$
The second derivative
[src]
20 / 2 2 \ 22*sin (x)*\- sin (x) + 21*cos (x)/
$$22 \left(- \sin^{2}{\left(x \right)} + 21 \cos^{2}{\left(x \right)}\right) \sin^{20}{\left(x \right)}$$
The third derivative
[src]
19 / 2 2 \ 88*sin (x)*\- 16*sin (x) + 105*cos (x)/*cos(x)
$$88 \left(- 16 \sin^{2}{\left(x \right)} + 105 \cos^{2}{\left(x \right)}\right) \sin^{19}{\left(x \right)} \cos{\left(x \right)}$$
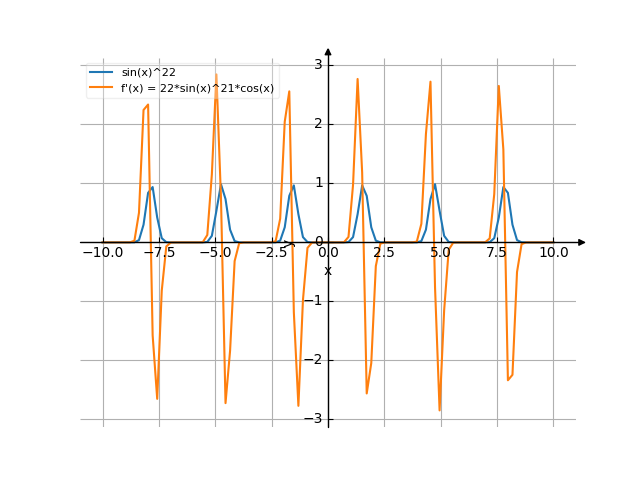
The graph