Integral of e^(-x)*cos(x)*dx dx
The solution
Detail solution
-
Use integration by parts, noting that the integrand eventually repeats itself.
-
For the integrand e−xcos(x)1:
Let u(x)=cos(x) and let dv(x)=e−x.
Then ∫e−xcos(x)1dx=−∫e−xsin(x)dx−e−xcos(x).
-
For the integrand e−xsin(x):
Let u(x)=sin(x) and let dv(x)=e−x.
Then ∫e−xcos(x)1dx=∫(−e−xcos(x))dx+e−xsin(x)−e−xcos(x).
-
Notice that the integrand has repeated itself, so move it to one side:
2∫e−xcos(x)1dx=e−xsin(x)−e−xcos(x)
Therefore,
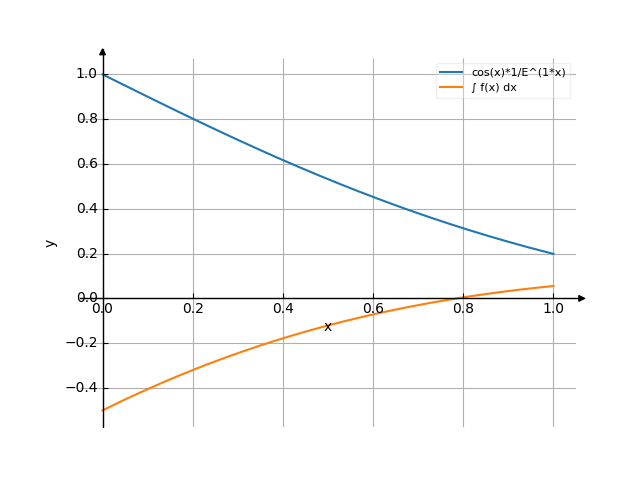
∫e−xcos(x)1dx=2e−xsin(x)−2e−xcos(x)
-
Now simplify:
−22e−xcos(x+4π)
-
Add the constant of integration:
−22e−xcos(x+4π)+constant
The answer is:
−22e−xcos(x+4π)+constant
The answer (Indefinite)
[src]
/
| -x -x
| -x e *sin(x) cos(x)*e
| e *cos(x)*1 dx = C + ---------- - ----------
| 2 2
/
2e−x(sinx−cosx)
The graph
-1 -1
1 e *sin(1) cos(1)*e
- + ---------- - ----------
2 2 2
2e−1(sin1−cos1)+21
=
-1 -1
1 e *sin(1) cos(1)*e
- + ---------- - ----------
2 2 2
−2ecos(1)+2esin(1)+21
Use the examples entering the upper and lower limits of integration.

![Find the integral of y = f(x) = e^(-x)*cos(x)*dx (e to the power of (minus x) multiply by co sinus of e of (x) multiply by dx) - with detailed solution [THERE'S THE ANSWER!] e^(-x)*cos(x)*dx](/media/krcore-image-pods/176/hash/indefinite/b/c9/c4ed18b9c1d04af64bdc761d5ba22.png)
 Integral of e^(-x)*cos(x)*dx
Integral of e^(-x)*cos(x)*dx
 Integral of sin(30)
Integral of sin(30)
 Integral of e^(-2x^2)
Integral of e^(-2x^2)
 Integral of e^(-2x)*sin(3x)
Integral of e^(-2x)*sin(3x)