x^3=125 equation
The teacher will be very surprised to see your correct solution 😉
The solution
Detail solution
Given the equation
Because equation degree is equal to = 3 - does not contain even numbers in the numerator, then
the equation has single real root.
Get the root 3-th degree of the equation sides:
We get:
or
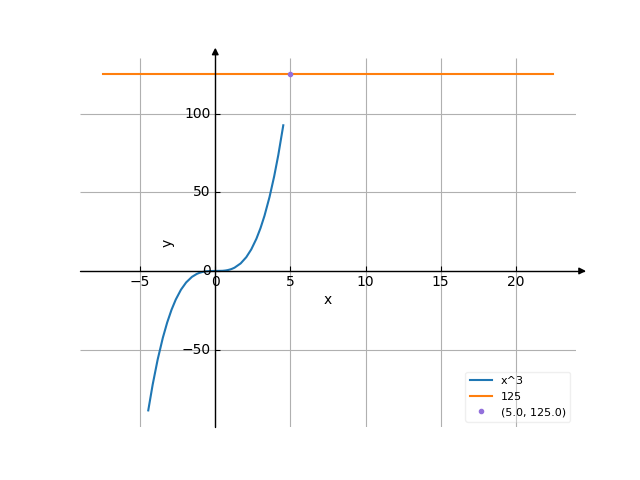
We get the answer: x = 5
All other 2 root(s) is the complex numbers.
do replacement:
then the equation will be the:
Any complex number can presented so:
substitute to the equation
where
- the magnitude of the complex number
Substitute r:
Using Euler’s formula, we find roots for p
so
and
then
where N=0,1,2,3,...
Looping through the values of N and substituting p into the formula for z
Consequently, the solution will be for z:
do backward replacement
The final answer:
Because equation degree is equal to = 3 - does not contain even numbers in the numerator, then
the equation has single real root.
Get the root 3-th degree of the equation sides:
We get:
or
We get the answer: x = 5
All other 2 root(s) is the complex numbers.
do replacement:
then the equation will be the:
Any complex number can presented so:
substitute to the equation
where
- the magnitude of the complex number
Substitute r:
Using Euler’s formula, we find roots for p
so
and
then
where N=0,1,2,3,...
Looping through the values of N and substituting p into the formula for z
Consequently, the solution will be for z:
do backward replacement
The final answer:
Vieta's Theorem
it is reduced cubic equation
where
Vieta Formulas
where
Vieta Formulas
Rapid solution
[src]
x_1 = 5
___
5 5*I*\/ 3
x_2 = - - - ---------
2 2
___
5 5*I*\/ 3
x_3 = - - + ---------
2 2
Sum and product of roots
[src]
sum
___ ___
5 5*I*\/ 3 5 5*I*\/ 3
5 + - - - --------- + - - + ---------
2 2 2 2
=
0
product
___ ___
5 5*I*\/ 3 5 5*I*\/ 3
5 * - - - --------- * - - + ---------
2 2 2 2
=
125
Numerical answer
[src]
x1 = 5.0
x2 = -2.5 - 4.33012701892219*i
x3 = -2.5 + 4.33012701892219*i
x3 = -2.5 + 4.33012701892219*i
The graph

![Solve the equation x³=125 (x cubed equally 125) - Find the roots of the equation in detail step by step. [THERE'S THE ANSWER!] x^3=125](/media/krcore-image-pods/176/hash/equation/c/20/028aba3bdbc5c32e3c886c7413274.png)
 Equation x^3=125
Equation x^3=125
 Equation 5x-25+2x²=17+13x
Equation 5x-25+2x²=17+13x
 Equation log2(x^2+3x)=2
Equation log2(x^2+3x)=2
 x^3
x^3
 x^3
x^3
 125
125