cos^3x equation
The teacher will be very surprised to see your correct solution 😉
The solution
Detail solution
Given the equation
transform
Do replacement
Given the equation
so
We get the answer: w = 0
do backward replacement
Given the equation
- this is the simplest trigonometric equation
This equation is transformed to
Or
, where n - is a integer
substitute w:
transform
Do replacement
Given the equation
so
We get the answer: w = 0
do backward replacement
Given the equation
- this is the simplest trigonometric equation
This equation is transformed to
Or
, where n - is a integer
substitute w:
Sum and product of roots
[src]
sum
pi 3*pi -- + ---- 2 2
=
2*pi
product
pi 3*pi --*---- 2 2
=
2 3*pi ----- 4
3*pi^2/4
Numerical answer
[src]
x1 = 92.6768935770301
x2 = 23.5620444336803
x3 = 95.818627417042
x4 = -14.1371260033657
x5 = -73.827410994311
x6 = -64.4025554047934
x7 = 73.8274768053124
x8 = -83.2523004207065
x9 = 7.85402475701276
x10 = 20.4203112367381
x11 = -42.411405413931
x12 = -23.5619897288019
x13 = 1.5708945053691
x14 = -45.5531401844306
x15 = 51.8363261592826
x16 = 42.4114617473496
x17 = -36.1282768063468
x18 = -95.8185603030962
x19 = 26.7034598912501
x20 = 29.8451754771722
x21 = 48.6946439323886
x22 = 23.5619763533234
x23 = 45.553194340988
x24 = -86.3937054164085
x25 = 70.6857435758276
x26 = -29.8451152214988
x27 = 36.128317789764
x28 = -89.5354410428862
x29 = -61.2611644481175
x30 = 64.4026122770508
x31 = 92.6770059000324
x32 = -80.1105785507599
x33 = -61.2611560468397
x34 = -39.2700061565569
x35 = -58.1194276545353
x36 = -67.5442906223714
x37 = 58.1194603256925
x38 = 67.5443442271897
x39 = -7.85396939058216
x40 = 67.5443333859623
x41 = 70.6858302611407
x42 = 48.6945935926021
x43 = 4.71228651848371
x44 = -1.57083925518957
x45 = 26.7034436275456
x46 = -17.2788562472482
x47 = 89.5354940921686
x48 = 86.3937628262857
x49 = -51.8362625267018
x50 = -20.4202554438585
x51 = -20.4203505482106
x52 = -42.4114638604687
x53 = -92.6770895717702
x54 = 1.57080273224359
x55 = 80.1106035284868
x56 = -83.2523059178598
x57 = 4.71229368085888
x58 = 45.5531567451367
x59 = 14.1371748405436
x59 = 14.1371748405436

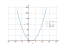 Equation k^2-4*k+3=0
Equation k^2-4*k+3=0
 cos^3x
cos^3x
 cos^3x
cos^3x