Mister Exam

Other calculators

y=ln(1+arctg^2x)
The derivative of the function/ y=ln(1+arctg^2x)

Derivative of y=ln(1+arctg^2x)

Function f() - derivative -N order at the point
v

The graph:

from to

Piecewise:

The solution

You have entered [src] 
   /        2   \
log\1 + atan (x)/
log⁡(atan⁡2(x)+1)\log{\left(\operatorname{atan}^{2}{\left(x \right)} + 1 \right)} 
d /   /        2   \\
--\log\1 + atan (x)//
dx
ddxlog⁡(atan⁡2(x)+1)\frac{d}{d x} \log{\left(\operatorname{atan}^{2}{\left(x \right)} + 1 \right)}
Transform
The graph
02468-8-6-4-2-10102-2
Plot the graph f(x)
Plot the graph f'(x)
The first derivative [src] 
       2*atan(x)       
-----------------------
/     2\ /        2   \
\1 + x /*\1 + atan (x)/
2atan⁡(x)(x2+1)(atan⁡2(x)+1)\frac{2 \operatorname{atan}{\left(x \right)}}{\left(x^{2} + 1\right) \left(\operatorname{atan}^{2}{\left(x \right)} + 1\right)}
Simplify
The second derivative [src] 
  /                         2    \
  |                   2*atan (x) |
2*|1 - 2*x*atan(x) - ------------|
  |                          2   |
  \                  1 + atan (x)/
----------------------------------
             2                    
     /     2\  /        2   \     
     \1 + x / *\1 + atan (x)/
2(−2xatan⁡(x)+1−2atan⁡2(x)atan⁡2(x)+1)(x2+1)2(atan⁡2(x)+1)\frac{2 \left(- 2 x \operatorname{atan}{\left(x \right)} + 1 - \frac{2 \operatorname{atan}^{2}{\left(x \right)}}{\operatorname{atan}^{2}{\left(x \right)} + 1}\right)}{\left(x^{2} + 1\right)^{2} \left(\operatorname{atan}^{2}{\left(x \right)} + 1\right)}
Simplify
The third derivative [src] 
  /                                                 2                        3                           2        \
  |            3*x            3*atan(x)          4*x *atan(x)          4*atan (x)                6*x*atan (x)     |
4*|-atan(x) - ------ - ----------------------- + ------------ + ------------------------ + -----------------------|
  |                2   /     2\ /        2   \           2                             2   /     2\ /        2   \|
  |           1 + x    \1 + x /*\1 + atan (x)/      1 + x       /     2\ /        2   \    \1 + x /*\1 + atan (x)/|
  \                                                             \1 + x /*\1 + atan (x)/                           /
-------------------------------------------------------------------------------------------------------------------
                                                      2                                                            
                                              /     2\  /        2   \                                             
                                              \1 + x / *\1 + atan (x)/
4⋅(4x2atan⁡(x)x2+1−3xx2+1+6xatan⁡2(x)(x2+1)(atan⁡2(x)+1)−atan⁡(x)−3atan⁡(x)(x2+1)(atan⁡2(x)+1)+4atan⁡3(x)(x2+1)(atan⁡2(x)+1)2)(x2+1)2(atan⁡2(x)+1)\frac{4 \cdot \left(\frac{4 x^{2} \operatorname{atan}{\left(x \right)}}{x^{2} + 1} - \frac{3 x}{x^{2} + 1} + \frac{6 x \operatorname{atan}^{2}{\left(x \right)}}{\left(x^{2} + 1\right) \left(\operatorname{atan}^{2}{\left(x \right)} + 1\right)} - \operatorname{atan}{\left(x \right)} - \frac{3 \operatorname{atan}{\left(x \right)}}{\left(x^{2} + 1\right) \left(\operatorname{atan}^{2}{\left(x \right)} + 1\right)} + \frac{4 \operatorname{atan}^{3}{\left(x \right)}}{\left(x^{2} + 1\right) \left(\operatorname{atan}^{2}{\left(x \right)} + 1\right)^{2}}\right)}{\left(x^{2} + 1\right)^{2} \left(\operatorname{atan}^{2}{\left(x \right)} + 1\right)}
Simplify
The graph
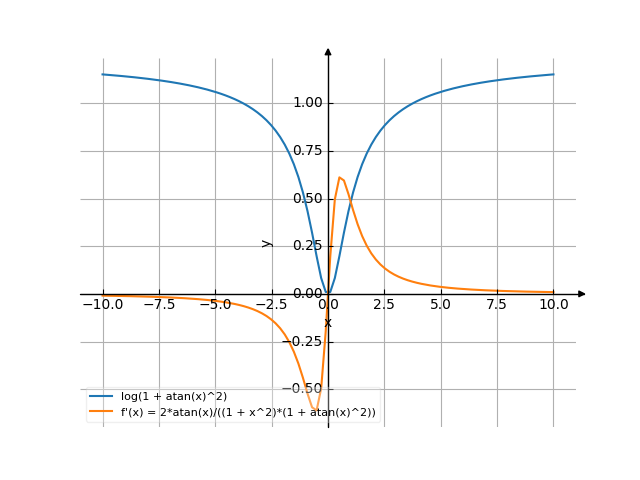
Derivative of y=ln(1+arctg^2x)
    © Mister Exam – Calculator