Mister Exam

Lang:

Other calculators

How to use it?
Derivative of:
Derivative of log(x)
Derivative of 2^x
Derivative of e^(3x)
Derivative of ln(x^3)
Integral of d{x}:
x^2sin(x^2)
Identical expressions
x^ two sin(x^2)
x squared sinus of (x squared )
x to the power of two sinus of (x squared )
x2sin(x2)
x2sinx2
x²sin(x²)
x to the power of 2sin(x to the power of 2)
x^2sinx^2
Similar expressions
(1-x^2)*sinx^2
(pi^2-x^2)(sin(x))^2
(-1)/(cos(x)^2*sin(x)^(2*x))
y=x^2sinx^2

The derivative of the function/ x^2sin(x^2)

Derivative of x^2sin(x^2)

The solution

You have entered [src]

 2    / 2\
x *sin\x /

$$x^{2} \sin{\left(x^{2} \right)}$$

d / 2    / 2\\
--\x *sin\x //
dx

$$\frac{d}{d x} x^{2} \sin{\left(x^{2} \right)}$$

Transform

Detail solution

Apply the product rule:

$\frac{d}{d x} f{\left(x \right)} g{\left(x \right)} = f{\left(x \right)} \frac{d}{d x} g{\left(x \right)} + g{\left(x \right)} \frac{d}{d x} f{\left(x \right)}$

$f{\left(x \right)} = x^{2}$ ; to find $\frac{d}{d x} f{\left(x \right)}$ :
1. Apply the power rule: $x^{2}$ goes to $2 x$
$g{\left(x \right)} = \sin{\left(x^{2} \right)}$ ; to find $\frac{d}{d x} g{\left(x \right)}$ :
1. Let $u = x^{2}$ .
2. The derivative of sine is cosine:
  
  $\frac{d}{d u} \sin{\left(u \right)} = \cos{\left(u \right)}$
3. Then, apply the chain rule. Multiply by $\frac{d}{d x} x^{2}$ :
  1. Apply the power rule: $x^{2}$ goes to $2 x$
  The result of the chain rule is:
  
  $2 x \cos{\left(x^{2} \right)}$
The result is: $2 x^{3} \cos{\left(x^{2} \right)} + 2 x \sin{\left(x^{2} \right)}$
Now simplify:

$2 x \left(x^{2} \cos{\left(x^{2} \right)} + \sin{\left(x^{2} \right)}\right)$

The answer is:

$2 x \left(x^{2} \cos{\left(x^{2} \right)} + \sin{\left(x^{2} \right)}\right)$

The graph

Plot the graph f(x)

Plot the graph f'(x)

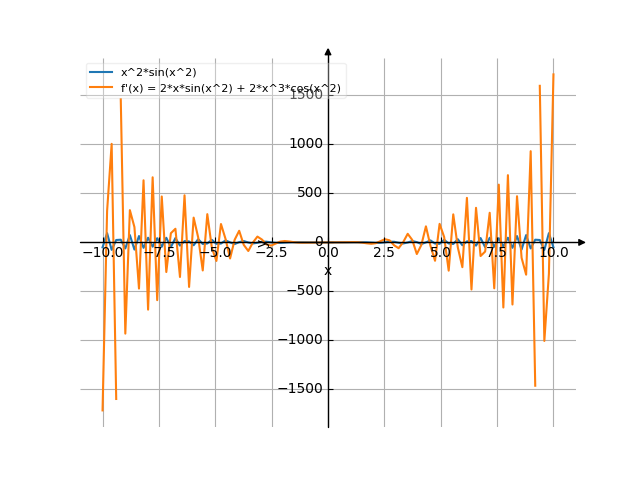
The first derivative [src]

       / 2\      3    / 2\
2*x*sin\x / + 2*x *cos\x /

$$2 x^{3} \cos{\left(x^{2} \right)} + 2 x \sin{\left(x^{2} \right)}$$

Simplify

The second derivative [src]

  /   2 /     / 2\      2    / 2\\      2    / 2\      / 2\\
2*\- x *\- cos\x / + 2*x *sin\x // + 4*x *cos\x / + sin\x //

$$2 \left(- x^{2} \cdot \left(2 x^{2} \sin{\left(x^{2} \right)} - \cos{\left(x^{2} \right)}\right) + 4 x^{2} \cos{\left(x^{2} \right)} + \sin{\left(x^{2} \right)}\right)$$

Simplify

The third derivative [src]

    /     / 2\    2 /     / 2\      2    / 2\\      2    / 2\\
4*x*\6*cos\x / - x *\3*sin\x / + 2*x *cos\x // - 6*x *sin\x //

$$4 x \left(- x^{2} \cdot \left(2 x^{2} \cos{\left(x^{2} \right)} + 3 \sin{\left(x^{2} \right)}\right) - 6 x^{2} \sin{\left(x^{2} \right)} + 6 \cos{\left(x^{2} \right)}\right)$$

Simplify

The graph

Other calculators

How to use it?

Identical expressions

Similar expressions

Derivative of x^2sin(x^2)

The graph:

Piecewise:

The solution