Mister Exam

Other calculators

1/((1+x^2)arctan^3x)

  • How to use it?

  • Integral of d{x}:
  • Integral of |-1+x|
  • Integral of -2x Integral of -2x
  • Integral of x*e^(-x)*dx Integral of x*e^(-x)*dx
  • Integral of k Integral of k

  • Identical expressions

  • one /((one +x^ two)arctan^3x)
  • 1 divide by ((1 plus x squared )arc tangent of cubed x)
  • one divide by ((one plus x to the power of two)arc tangent of cubed x)
  • 1/((1+x2)arctan3x)
  • 1/1+x2arctan3x
  • 1/((1+x²)arctan³x)
  • 1/((1+x to the power of 2)arctan to the power of 3x)
  • 1/1+x^2arctan^3x
  • 1 divide by ((1+x^2)arctan^3x)
  • 1/((1+x^2)arctan^3x)dx

  • Similar expressions

  • 1/((1-x^2)arctan^3x)
Solving integrals/ 1/((1+x^2)arctan^3x)

Integral of 1/((1+x^2)arctan^3x) dx

Limits of integration:

from to
v

The graph:

from to

Piecewise:

The solution

You have entered [src] 
  1                       
  /                       
 |                        
 |            1           
 |  1*----------------- dx
 |    /     2\     3      
 |    \1 + x /*atan (x)   
 |                        
/                         
0
$$\int\limits_{0}^{1} 1 \cdot \frac{1}{\left(x^{2} + 1\right) \operatorname{atan}^{3}{\left(x \right)}}\, dx$$ 
Integral(1/((1 + x^2)*atan(x)^3), (x, 0, 1))
Detail solution

    TrigSubstitutionRule(theta=_theta, func=tan(_theta), rewritten=atan(tan(_theta))**(-3), substep=URule(u_var=_u, u_func=atan(tan(_theta)), constant=1, substep=PowerRule(base=_u, exp=-3, context=_u**(-3), symbol=_u), context=atan(tan(_theta))**(-3), symbol=_theta), restriction=True, context=1/((x**2 + 1)*atan(x)**3), symbol=x)

  1. Add the constant of integration:

The answer is:

The answer (Indefinite) [src] 
  /                                       
 |                                        
 |           1                      1     
 | 1*----------------- dx = C - ----------
 |   /     2\     3                   2   
 |   \1 + x /*atan (x)          2*atan (x)
 |                                        
/
$$\int 1 \cdot \frac{1}{\left(x^{2} + 1\right) \operatorname{atan}^{3}{\left(x \right)}}\, dx = C - \frac{1}{2 \operatorname{atan}^{2}{\left(x \right)}}$$
Simplify
The graph
Plot the graph f(x)
The answer [src] 
oo
$$\infty$$ 
=
= 
oo
$$\infty$$
Упростить
Numerical answer [src] 
9.15365037903492e+37
9.15365037903492e+37
The graph
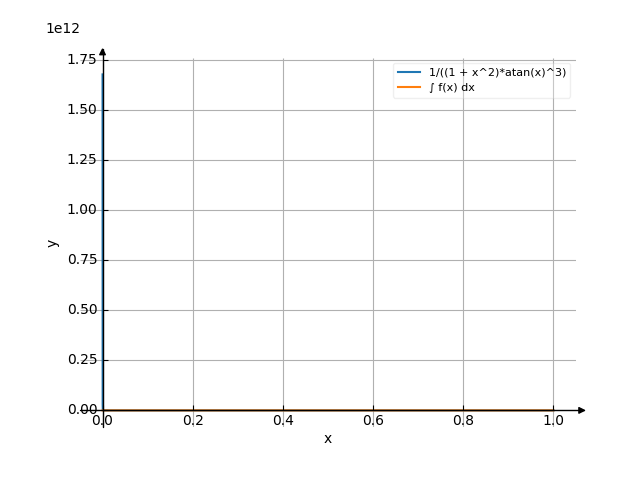
Integral of 1/((1+x^2)arctan^3x) dx

    Use the examples entering the upper and lower limits of integration.

    © Mister Exam – Calculator