x²+(x+2)²=10² equation
The teacher will be very surprised to see your correct solution 😉
The solution
Detail solution
Move right part of the equation to
left part with negative sign.
The equation is transformed from
to
Expand the expression in the equation
We get the quadratic equation
This equation is of the form
A quadratic equation can be solved using the discriminant
The roots of the quadratic equation:
where is the discriminant.
Because
, then
Because D > 0, then the equation has two roots.
or
Simplify
Simplify
left part with negative sign.
The equation is transformed from
to
Expand the expression in the equation
We get the quadratic equation
This equation is of the form
A quadratic equation can be solved using the discriminant
The roots of the quadratic equation:
where is the discriminant.
Because
, then
Because D > 0, then the equation has two roots.
or
Simplify
Simplify
The graph


![Solve the equation x²+(x+2)²=10² (x² plus (x plus 2)² equally 10²) - Find the roots of the equation in detail step by step. [THERE'S THE ANSWER!] x²+(x+2)²=10²](/media/krcore-image-pods/176/hash/equation/c/c7/94d96c5abb3d8ab6b4b15b6320e06.png)
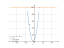 Equation x²+(x+2)²=10²
Equation x²+(x+2)²=10²
 Equation Sin^2x+5sinx+4=0
Equation Sin^2x+5sinx+4=0