Mister Exam
Other calculators
-
How to use it?
- Equation:
-
Equation (2x-4)(x-11)+28=0
-
Equation cos(x+pi/3)=0
- Equation x³-2x²+x=0
- Equation x:2,404=5,02
- Express {x} in terms of y where:
- 9*x-8*y=6
- 15*x+15*y=16
- -4*x+18*y=-14
- 8*x+7*y=-13
- Graphing y =:
-
cos(x+pi/3)
-
Identical expressions
- cos(x+pi/ three)= zero
- co sinus of e of (x plus Pi divide by 3) equally 0
- co sinus of e of (x plus Pi divide by three) equally zero
- cosx+pi/3=0
- cos(x+pi/3)=O
- cos(x+pi divide by 3)=0
-
Similar expressions
- cos(x+(pi/3))=1
- cos(x-pi/3)=0
-
What you mean?
- cos((x + pi)/3) = 0
- False
- False
You entered:
cos(x+pi/3)=0
What you mean?
cos(x+pi/3)=0 equation
The teacher will be very surprised to see your correct solution 😉
The solution
Detail solution
Given the equation
$$\cos{\left(x + \frac{\pi}{3} \right)} = 0$$
- this is the simplest trigonometric equation
We get:
$$\cos{\left(x + \frac{\pi}{3} \right)} = 0$$
This equation is transformed to
$$x + \frac{\pi}{3} = \pi n + \operatorname{acos}{\left(0 \right)}$$
$$x + \frac{\pi}{3} = \pi n - \pi + \operatorname{acos}{\left(0 \right)}$$
Or
$$x + \frac{\pi}{3} = \pi n + \frac{\pi}{2}$$
$$x + \frac{\pi}{3} = \pi n - \frac{\pi}{2}$$
, where n - is a integer
Move
$$\frac{\pi}{3}$$
to right part of the equation
with the opposite sign, in total:
$$x = \pi n + \frac{\pi}{6}$$
$$x = \pi n - \frac{5 \pi}{6}$$
$$\cos{\left(x + \frac{\pi}{3} \right)} = 0$$
- this is the simplest trigonometric equation
with the change of sign in 0
We get:
$$\cos{\left(x + \frac{\pi}{3} \right)} = 0$$
This equation is transformed to
$$x + \frac{\pi}{3} = \pi n + \operatorname{acos}{\left(0 \right)}$$
$$x + \frac{\pi}{3} = \pi n - \pi + \operatorname{acos}{\left(0 \right)}$$
Or
$$x + \frac{\pi}{3} = \pi n + \frac{\pi}{2}$$
$$x + \frac{\pi}{3} = \pi n - \frac{\pi}{2}$$
, where n - is a integer
Move
$$\frac{\pi}{3}$$
to right part of the equation
with the opposite sign, in total:
$$x = \pi n + \frac{\pi}{6}$$
$$x = \pi n - \frac{5 \pi}{6}$$
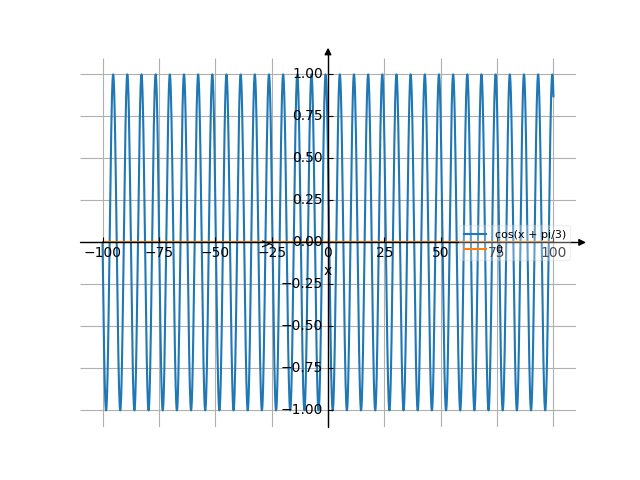
The graph