Mister Exam

Other calculators

y=x⁸/x²
The derivative of the function/ y=x⁸/x²

You entered:

y=x⁸/x²

What you mean?

x^8/(x^2)
 
x^((8/x)^2)
 
x^((8/x)^2)

Derivative of y=x⁸/x²

Function f() - derivative -N order at the point
v

The graph:

from to

Piecewise:

The solution

You have entered [src] 
 8
x 
--
 2
x
x8x2\frac{x^{8}}{x^{2}} 
  / 8\
d |x |
--|--|
dx| 2|
  \x /
ddxx8x2\frac{d}{d x} \frac{x^{8}}{x^{2}}
Transform
Detail solution

  1. Apply the quotient rule, which is:

    ddxf(x)g(x)=−f(x)ddxg(x)+g(x)ddxf(x)g2(x)\frac{d}{d x} \frac{f{\left(x \right)}}{g{\left(x \right)}} = \frac{- f{\left(x \right)} \frac{d}{d x} g{\left(x \right)} + g{\left(x \right)} \frac{d}{d x} f{\left(x \right)}}{g^{2}{\left(x \right)}}

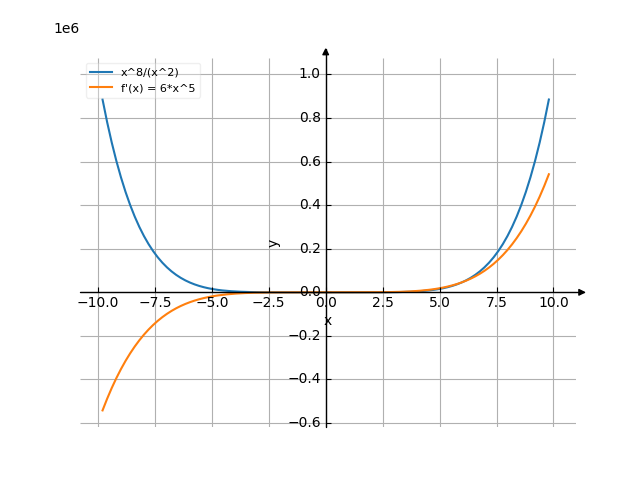
    f(x)=x8f{\left(x \right)} = x^{8} and g(x)=x2g{\left(x \right)} = x^{2}.

    To find ddxf(x)\frac{d}{d x} f{\left(x \right)}:

    1. Apply the power rule: x8x^{8} goes to 8x78 x^{7}

    To find ddxg(x)\frac{d}{d x} g{\left(x \right)}:

    1. Apply the power rule: x2x^{2} goes to 2x2 x

    Now plug in to the quotient rule:

    6x56 x^{5}

The answer is:

6x56 x^{5}

The graph
02468-8-6-4-2-1010-20000002000000
Plot the graph f(x)
Plot the graph f'(x)
The first derivative [src] 
            7
     5   8*x 
- 2*x  + ----
           2 
          x
8x7x2−2x5\frac{8 x^{7}}{x^{2}} - 2 x^{5}
Simplify
The second derivative [src] 
    4
30*x
30x430 x^{4}
Simplify
The third derivative [src] 
     3
120*x
120x3120 x^{3}
Simplify
The graph
Derivative of y=x⁸/x²
    © Mister Exam – Calculator