Mister Exam
Other calculators
- Derivative of a implicit defined function
- Derivative of Parametric Function
- Partial derivative of the function
- Curve tracing functions Step by Step
- Integral Step by Step
- Differential equations Step by Step
- Limits Step by Step
-
How to use it?
- Derivative of:
-
Derivative of tg4x
- Derivative of factorial(x)
-
Derivative of e^(2x+1)
-
Derivative of y=tanh^-1(1-2x)
-
Identical expressions
- y=tanh^- one (one -2x)
- y equally hyperbolic tangent of gent of to the power of minus 1(1 minus 2x)
- y equally hyperbolic tangent of gent of to the power of minus one (one minus 2x)
- y=tanh-1(1-2x)
- y=tanh-11-2x
- y=tanh^-11-2x
-
Similar expressions
- y=tanh^+1(1-2x)
- y=tanh^-1(1+2x)
Derivative of y=tanh^-1(1-2x)
The solution
You have entered
[src]
1 ------------- tanh(1 - 2*x)
$$\frac{1}{\tanh{\left(- 2 x + 1 \right)}}$$
d / 1 \ --|-------------| dx\tanh(1 - 2*x)/
$$\frac{d}{d x} \frac{1}{\tanh{\left(- 2 x + 1 \right)}}$$
The first derivative
[src]
2
2 - 2*tanh (-1 + 2*x)
---------------------
2
tanh (1 - 2*x)
$$\frac{- 2 \tanh^{2}{\left(2 x - 1 \right)} + 2}{\tanh^{2}{\left(- 2 x + 1 \right)}}$$
The second derivative
[src]
/ 2 \
| -1 + tanh (-1 + 2*x)| / 2 \
8*|1 - --------------------|*\-1 + tanh (-1 + 2*x)/
| 2 |
\ tanh (-1 + 2*x) /
---------------------------------------------------
tanh(-1 + 2*x)
$$\frac{8 \left(1 - \frac{\tanh^{2}{\left(2 x - 1 \right)} - 1}{\tanh^{2}{\left(2 x - 1 \right)}}\right) \left(\tanh^{2}{\left(2 x - 1 \right)} - 1\right)}{\tanh{\left(2 x - 1 \right)}}$$
The third derivative
[src]
/ 3 2\ | / 2 \ / 2 \ | | 2 3*\-1 + tanh (-1 + 2*x)/ 5*\-1 + tanh (-1 + 2*x)/ | 16*|2 - 2*tanh (-1 + 2*x) - ------------------------- + -------------------------| | 4 2 | \ tanh (-1 + 2*x) tanh (-1 + 2*x) /
$$16 \left(- 2 \tanh^{2}{\left(2 x - 1 \right)} + \frac{5 \left(\tanh^{2}{\left(2 x - 1 \right)} - 1\right)^{2}}{\tanh^{2}{\left(2 x - 1 \right)}} + 2 - \frac{3 \left(\tanh^{2}{\left(2 x - 1 \right)} - 1\right)^{3}}{\tanh^{4}{\left(2 x - 1 \right)}}\right)$$
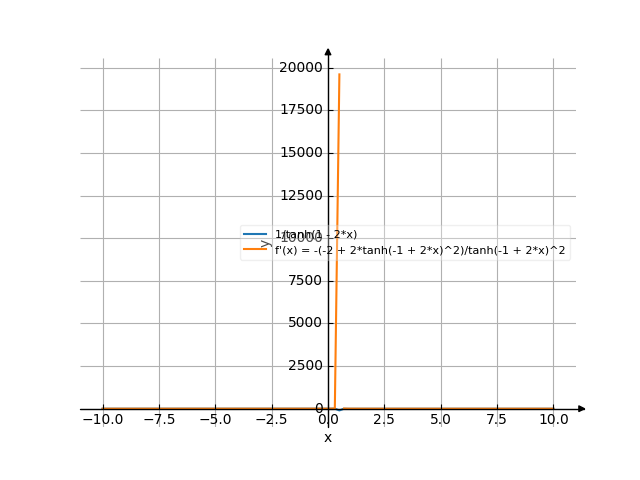
The graph