Mister Exam

Other calculators

arctg((tgx-ctgx)/sqrt(2))
The derivative of the function/ arctg((tgx-ctgx)/sqrt(2))

Derivative of arctg((tgx-ctgx)/sqrt(2))

Function f() - derivative -N order at the point
v

The graph:

from to

Piecewise:

The solution

You have entered [src] 
    /tan(x) - cot(x)\
atan|---------------|
    |       ___     |
    \     \/ 2      /
atan⁡(tan⁡(x)−cot⁡(x)2)\operatorname{atan}{\left(\frac{\tan{\left(x \right)} - \cot{\left(x \right)}}{\sqrt{2}} \right)} 
d /    /tan(x) - cot(x)\\
--|atan|---------------||
dx|    |       ___     ||
  \    \     \/ 2      //
ddxatan⁡(tan⁡(x)−cot⁡(x)2)\frac{d}{d x} \operatorname{atan}{\left(\frac{\tan{\left(x \right)} - \cot{\left(x \right)}}{\sqrt{2}} \right)}
Transform
The graph
02468-8-6-4-2-10105-5
Plot the graph f(x)
Plot the graph f'(x)
The first derivative [src] 
  ___ /       2         2   \
\/ 2 *\2 + cot (x) + tan (x)/
-----------------------------
    /                     2\ 
    |    (tan(x) - cot(x)) | 
  2*|1 + ------------------| 
    \            2         /
2(tan⁡2(x)+cot⁡2(x)+2)2((tan⁡(x)−cot⁡(x))22+1)\frac{\sqrt{2} \left(\tan^{2}{\left(x \right)} + \cot^{2}{\left(x \right)} + 2\right)}{2 \left(\frac{\left(\tan{\left(x \right)} - \cot{\left(x \right)}\right)^{2}}{2} + 1\right)}
Simplify
The second derivative [src] 
        /                                                                     2                   \
        |                                              /       2         2   \                    |
    ___ |/       2   \          /       2   \          \2 + cot (x) + tan (x)/ *(-cot(x) + tan(x))|
2*\/ 2 *|\1 + tan (x)/*tan(x) - \1 + cot (x)/*cot(x) - -------------------------------------------|
        |                                                                              2          |
        \                                                        2 + (-cot(x) + tan(x))           /
---------------------------------------------------------------------------------------------------
                                                            2                                      
                                      2 + (-cot(x) + tan(x))
22((tan⁡2(x)+1)tan⁡(x)−(cot⁡2(x)+1)cot⁡(x)−(tan⁡(x)−cot⁡(x))(tan⁡2(x)+cot⁡2(x)+2)2(tan⁡(x)−cot⁡(x))2+2)(tan⁡(x)−cot⁡(x))2+2\frac{2 \sqrt{2} \left(\left(\tan^{2}{\left(x \right)} + 1\right) \tan{\left(x \right)} - \left(\cot^{2}{\left(x \right)} + 1\right) \cot{\left(x \right)} - \frac{\left(\tan{\left(x \right)} - \cot{\left(x \right)}\right) \left(\tan^{2}{\left(x \right)} + \cot^{2}{\left(x \right)} + 2\right)^{2}}{\left(\tan{\left(x \right)} - \cot{\left(x \right)}\right)^{2} + 2}\right)}{\left(\tan{\left(x \right)} - \cot{\left(x \right)}\right)^{2} + 2}
Simplify
The third derivative [src] 
        /                                                         3                                                                                                    3                                                                                             \
        |             2                2   /       2         2   \                                                                            2 /       2         2   \                         //       2   \          /       2   \       \ /       2         2   \|
    ___ |/       2   \    /       2   \    \2 + cot (x) + tan (x)/         2    /       2   \        2    /       2   \   4*(-cot(x) + tan(x)) *\2 + cot (x) + tan (x)/    6*(-cot(x) + tan(x))*\\1 + tan (x)/*tan(x) - \1 + cot (x)/*cot(x)/*\2 + cot (x) + tan (x)/|
2*\/ 2 *|\1 + cot (x)/  + \1 + tan (x)/  - ------------------------ + 2*cot (x)*\1 + cot (x)/ + 2*tan (x)*\1 + tan (x)/ + ---------------------------------------------- - ------------------------------------------------------------------------------------------|
        |                                                        2                                                                                           2                                                                    2                                  |
        |                                  2 + (-cot(x) + tan(x))                                                                   /                      2\                                               2 + (-cot(x) + tan(x))                                   |
        \                                                                                                                           \2 + (-cot(x) + tan(x)) /                                                                                                        /
----------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
                                                                                                                                             2                                                                                                                        
                                                                                                                       2 + (-cot(x) + tan(x))
22(2(tan⁡2(x)+1)tan⁡2(x)+2(cot⁡2(x)+1)cot⁡2(x)+4(tan⁡(x)−cot⁡(x))2(tan⁡2(x)+cot⁡2(x)+2)3((tan⁡(x)−cot⁡(x))2+2)2−6((tan⁡2(x)+1)tan⁡(x)−(cot⁡2(x)+1)cot⁡(x))(tan⁡(x)−cot⁡(x))(tan⁡2(x)+cot⁡2(x)+2)(tan⁡(x)−cot⁡(x))2+2+(tan⁡2(x)+1)2+(cot⁡2(x)+1)2−(tan⁡2(x)+cot⁡2(x)+2)3(tan⁡(x)−cot⁡(x))2+2)(tan⁡(x)−cot⁡(x))2+2\frac{2 \sqrt{2} \left(2 \left(\tan^{2}{\left(x \right)} + 1\right) \tan^{2}{\left(x \right)} + 2 \left(\cot^{2}{\left(x \right)} + 1\right) \cot^{2}{\left(x \right)} + \frac{4 \left(\tan{\left(x \right)} - \cot{\left(x \right)}\right)^{2} \left(\tan^{2}{\left(x \right)} + \cot^{2}{\left(x \right)} + 2\right)^{3}}{\left(\left(\tan{\left(x \right)} - \cot{\left(x \right)}\right)^{2} + 2\right)^{2}} - \frac{6 \left(\left(\tan^{2}{\left(x \right)} + 1\right) \tan{\left(x \right)} - \left(\cot^{2}{\left(x \right)} + 1\right) \cot{\left(x \right)}\right) \left(\tan{\left(x \right)} - \cot{\left(x \right)}\right) \left(\tan^{2}{\left(x \right)} + \cot^{2}{\left(x \right)} + 2\right)}{\left(\tan{\left(x \right)} - \cot{\left(x \right)}\right)^{2} + 2} + \left(\tan^{2}{\left(x \right)} + 1\right)^{2} + \left(\cot^{2}{\left(x \right)} + 1\right)^{2} - \frac{\left(\tan^{2}{\left(x \right)} + \cot^{2}{\left(x \right)} + 2\right)^{3}}{\left(\tan{\left(x \right)} - \cot{\left(x \right)}\right)^{2} + 2}\right)}{\left(\tan{\left(x \right)} - \cot{\left(x \right)}\right)^{2} + 2}
Simplify
The graph
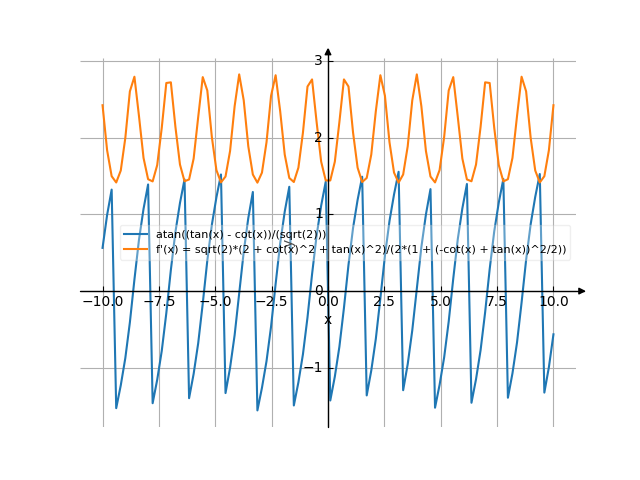
Derivative of arctg((tgx-ctgx)/sqrt(2))
    © Mister Exam – Calculator