Mister Exam

Other calculators

arcsinx^2+arccos2x.
The derivative of the function/ arcsinx^2+arccos2x.

Derivative of arcsinx^2+arccos2x.

Function f() - derivative -N order at the point
v

The graph:

from to

Piecewise:

The solution

You have entered [src] 
    2               
asin (x) + acos(2*x)
asin⁡2(x)+acos⁡(2x)\operatorname{asin}^{2}{\left(x \right)} + \operatorname{acos}{\left(2 x \right)} 
d /    2               \
--\asin (x) + acos(2*x)/
dx
ddx(asin⁡2(x)+acos⁡(2x))\frac{d}{d x} \left(\operatorname{asin}^{2}{\left(x \right)} + \operatorname{acos}{\left(2 x \right)}\right)
Transform
The graph
02468-8-6-4-2-10105-5
Plot the graph f(x)
Plot the graph f'(x)
The first derivative [src] 
        2          2*asin(x) 
- ------------- + -----------
     __________      ________
    /        2      /      2 
  \/  1 - 4*x     \/  1 - x
2asin⁡(x)−x2+1−2−4x2+1\frac{2 \operatorname{asin}{\left(x \right)}}{\sqrt{- x^{2} + 1}} - \frac{2}{\sqrt{- 4 x^{2} + 1}}
Simplify
The second derivative [src] 
  /     1           4*x         x*asin(x) \
2*|- ------- - ------------- + -----------|
  |        2             3/2           3/2|
  |  -1 + x    /       2\      /     2\   |
  \            \1 - 4*x /      \1 - x /   /
2(xasin⁡(x)(−x2+1)32−1x2−1−4x(−4x2+1)32)2 \left(\frac{x \operatorname{asin}{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}}} - \frac{1}{x^{2} - 1} - \frac{4 x}{\left(- 4 x^{2} + 1\right)^{\frac{3}{2}}}\right)
Simplify
The third derivative [src] 
  /                                        2                       2        \
  |        4           asin(x)         48*x           3*x       3*x *asin(x)|
2*|- ------------- + ----------- - ------------- + ---------- + ------------|
  |            3/2           3/2             5/2            2           5/2 |
  |  /       2\      /     2\      /       2\      /      2\    /     2\    |
  \  \1 - 4*x /      \1 - x /      \1 - 4*x /      \-1 + x /    \1 - x /    /
2⋅(3x(x2−1)2+3x2asin⁡(x)(−x2+1)52+asin⁡(x)(−x2+1)32−48x2(−4x2+1)52−4(−4x2+1)32)2 \cdot \left(\frac{3 x}{\left(x^{2} - 1\right)^{2}} + \frac{3 x^{2} \operatorname{asin}{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{5}{2}}} + \frac{\operatorname{asin}{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}}} - \frac{48 x^{2}}{\left(- 4 x^{2} + 1\right)^{\frac{5}{2}}} - \frac{4}{\left(- 4 x^{2} + 1\right)^{\frac{3}{2}}}\right)
Simplify
The graph
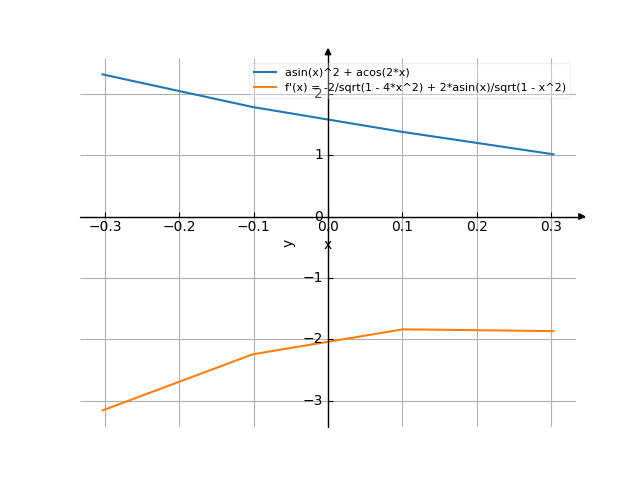
Derivative of arcsinx^2+arccos2x.
    © Mister Exam – Calculator