Derivative of arcsin*sqrt(4-5x)
The solution
You have entered
[src]
_________ asin(x)*\/ 4 - 5*x
$$\sqrt{4 - 5 x} \operatorname{asin}{\left(x \right)}$$
d / _________\ --\asin(x)*\/ 4 - 5*x / dx
$$\frac{d}{d x} \sqrt{4 - 5 x} \operatorname{asin}{\left(x \right)}$$
The first derivative
[src]
_________ \/ 4 - 5*x 5*asin(x) ----------- - ------------- ________ _________ / 2 2*\/ 4 - 5*x \/ 1 - x
$$- \frac{5 \operatorname{asin}{\left(x \right)}}{2 \sqrt{4 - 5 x}} + \frac{\sqrt{4 - 5 x}}{\sqrt{1 - x^{2}}}$$
The second derivative
[src]
_________
5 25*asin(x) x*\/ 4 - 5*x
- ----------------------- - -------------- + -------------
________ 3/2 3/2
/ 2 _________ 4*(4 - 5*x) / 2\
\/ 1 - x *\/ 4 - 5*x \1 - x /
$$\frac{x \sqrt{4 - 5 x}}{\left(1 - x^{2}\right)^{\frac{3}{2}}} - \frac{25 \operatorname{asin}{\left(x \right)}}{4 \left(4 - 5 x\right)^{\frac{3}{2}}} - \frac{5}{\sqrt{1 - x^{2}} \sqrt{4 - 5 x}}$$
The third derivative
[src]
/ / 2 \ \ | _________ | 3*x | | | \/ 4 - 5*x *|-1 + -------| | | | 2| | | 75 375*asin(x) \ -1 + x / 15*x | -|-------------------------- + -------------- + -------------------------- + -------------------------| | ________ 5/2 3/2 3/2 | | / 2 3/2 8*(4 - 5*x) / 2\ / 2\ _________| \4*\/ 1 - x *(4 - 5*x) \1 - x / 2*\1 - x / *\/ 4 - 5*x /
$$- (\frac{15 x}{2 \left(1 - x^{2}\right)^{\frac{3}{2}} \sqrt{4 - 5 x}} + \frac{375 \operatorname{asin}{\left(x \right)}}{8 \left(4 - 5 x\right)^{\frac{5}{2}}} + \frac{75}{4 \sqrt{1 - x^{2}} \left(4 - 5 x\right)^{\frac{3}{2}}} + \frac{\sqrt{4 - 5 x} \left(\frac{3 x^{2}}{x^{2} - 1} - 1\right)}{\left(1 - x^{2}\right)^{\frac{3}{2}}})$$
The graph
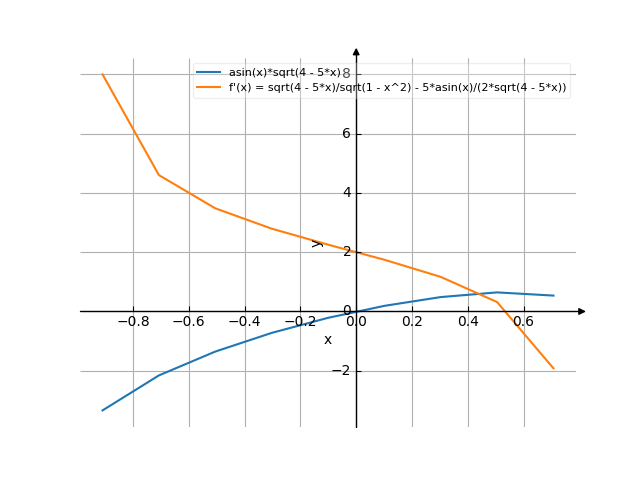

![Find the derivative of y' = f'(x) = arcsin*sqrt(4-5x) (arc sinus of multiply by square root of (4 minus 5x)) - functions. Find the derivative of the function at the point. [THERE'S THE ANSWER!] arcsin*sqrt(4-5x)](/media/krcore-image-pods/176/hash/derivative/0/d7/4a8c47c00f613b2be2ac95f939b2f.png)
 Derivative of x^12
Derivative of x^12
 Derivative of (2*e)^cos(log(6*x-1))^(2)
Derivative of (2*e)^cos(log(6*x-1))^(2)
 Derivative of 1/tg(x)
Derivative of 1/tg(x)
 Derivative of 1/3*x
Derivative of 1/3*x