Mister Exam

Lang:

Other calculators

How to use it?
Equation:
Equation 16x-3=8x-43
Equation 6x-2x+1=5
Equation 2x-4=8+2x
Equation x^4-2*x^2-15=0
Express {x} in terms of y where:
-11*x+10*y=-3
-10*x+13*y=5
10*x-6*y=9
-11*x-11*y=4
Graphing y =:
x^6
Integral of d{x}:
x^6
Derivative of:
x^6
Identical expressions
x^ six =(6x- eight)^ three
x to the power of 6 equally (6x minus 8) cubed
x to the power of six equally (6x minus eight) to the power of three
x6=(6x-8)3
x6=6x-83
x⁶=(6x-8)³
x to the power of 6=(6x-8) to the power of 3
x^6=6x-8^3
Similar expressions
x^6=(6x+8)^3

x^6=(6x-8)^3 equation

The teacher will be very surprised to see your correct solution 😉

The solution

You have entered [src]

 6            3
x  = (6*x - 8)

$$x^{6} = \left(6 x - 8\right)^{3}$$

Simplify

The graph

Plot the graph f1(x)

Plot the graph f2(x)

Rapid solution [src]

x_1 = 2

$$x_{1} = 2$$

Simplify

x_2 = 4

$$x_{2} = 4$$

Simplify

              /      ___              /    /     ___\\\              /    /     ___\\
        3     |  3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|
x_3 = - - + I*|- ------- + \/ 217 *cos|--------------|| + \/ 217 *sin|--------------|
        2     \     2                 \      2       //              \      2       /

$$x_{3} = - \frac{3}{2} + \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + i \left(- \frac{3 \sqrt{3}}{2} + \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)}\right)$$

Simplify

              /    ___              /    /     ___\\\              /    /     ___\\
        3     |3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|
x_4 = - - + I*|------- + \/ 217 *cos|--------------|| - \/ 217 *sin|--------------|
        2     \   2                 \      2       //              \      2       /

$$x_{4} = - \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3}{2} + i \left(\frac{3 \sqrt{3}}{2} + \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)}\right)$$

Simplify

              /    ___              /    /     ___\\\              /    /     ___\\
        3     |3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|
x_5 = - - + I*|------- - \/ 217 *cos|--------------|| + \/ 217 *sin|--------------|
        2     \   2                 \      2       //              \      2       /

$$x_{5} = - \frac{3}{2} + \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + i \left(- \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + \frac{3 \sqrt{3}}{2}\right)$$

Simplify

              /      ___              /    /     ___\\\              /    /     ___\\
        3     |  3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|
x_6 = - - + I*|- ------- - \/ 217 *cos|--------------|| - \/ 217 *sin|--------------|
        2     \     2                 \      2       //              \      2       /

$$x_{6} = - \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3}{2} + i \left(- \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3 \sqrt{3}}{2}\right)$$

Simplify

Sum and product of roots [src]

sum

                /      ___              /    /     ___\\\              /    /     ___\\           /    ___              /    /     ___\\\              /    /     ___\\           /    ___              /    /     ___\\\              /    /     ___\\           /      ___              /    /     ___\\\              /    /     ___\\
          3     |  3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|     3     |3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|     3     |3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|     3     |  3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|
2 + 4 + - - + I*|- ------- + \/ 217 *cos|--------------|| + \/ 217 *sin|--------------| + - - + I*|------- + \/ 217 *cos|--------------|| - \/ 217 *sin|--------------| + - - + I*|------- - \/ 217 *cos|--------------|| + \/ 217 *sin|--------------| + - - + I*|- ------- - \/ 217 *cos|--------------|| - \/ 217 *sin|--------------|
          2     \     2                 \      2       //              \      2       /     2     \   2                 \      2       //              \      2       /     2     \   2                 \      2       //              \      2       /     2     \     2                 \      2       //              \      2       /

$$\left(2\right) + \left(4\right) + \left(- \frac{3}{2} + \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + i \left(- \frac{3 \sqrt{3}}{2} + \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)}\right)\right) + \left(- \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3}{2} + i \left(\frac{3 \sqrt{3}}{2} + \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)}\right)\right) + \left(- \frac{3}{2} + \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + i \left(- \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + \frac{3 \sqrt{3}}{2}\right)\right) + \left(- \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3}{2} + i \left(- \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3 \sqrt{3}}{2}\right)\right)$$

  /      ___              /    /     ___\\\     /      ___              /    /     ___\\\     /    ___              /    /     ___\\\     /    ___              /    /     ___\\\
  |  3*\/ 3    4 _____    |atan\17*\/ 3 /||     |  3*\/ 3    4 _____    |atan\17*\/ 3 /||     |3*\/ 3    4 _____    |atan\17*\/ 3 /||     |3*\/ 3    4 _____    |atan\17*\/ 3 /||
I*|- ------- + \/ 217 *cos|--------------|| + I*|- ------- - \/ 217 *cos|--------------|| + I*|------- + \/ 217 *cos|--------------|| + I*|------- - \/ 217 *cos|--------------||
  \     2                 \      2       //     \     2                 \      2       //     \   2                 \      2       //     \   2                 \      2       //

$$i \left(- \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3 \sqrt{3}}{2}\right) + i \left(- \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + \frac{3 \sqrt{3}}{2}\right) + i \left(- \frac{3 \sqrt{3}}{2} + \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)}\right) + i \left(\frac{3 \sqrt{3}}{2} + \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)}\right)$$

Simplify

product

                /      ___              /    /     ___\\\              /    /     ___\\           /    ___              /    /     ___\\\              /    /     ___\\           /    ___              /    /     ___\\\              /    /     ___\\           /      ___              /    /     ___\\\              /    /     ___\\
          3     |  3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|     3     |3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|     3     |3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|     3     |  3*\/ 3    4 _____    |atan\17*\/ 3 /||   4 _____    |atan\17*\/ 3 /|
2 * 4 * - - + I*|- ------- + \/ 217 *cos|--------------|| + \/ 217 *sin|--------------| * - - + I*|------- + \/ 217 *cos|--------------|| - \/ 217 *sin|--------------| * - - + I*|------- - \/ 217 *cos|--------------|| + \/ 217 *sin|--------------| * - - + I*|- ------- - \/ 217 *cos|--------------|| - \/ 217 *sin|--------------|
          2     \     2                 \      2       //              \      2       /     2     \   2                 \      2       //              \      2       /     2     \   2                 \      2       //              \      2       /     2     \     2                 \      2       //              \      2       /

$$\left(2\right) * \left(4\right) * \left(- \frac{3}{2} + \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + i \left(- \frac{3 \sqrt{3}}{2} + \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)}\right)\right) * \left(- \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3}{2} + i \left(\frac{3 \sqrt{3}}{2} + \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)}\right)\right) * \left(- \frac{3}{2} + \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + i \left(- \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} + \frac{3 \sqrt{3}}{2}\right)\right) * \left(- \sqrt[4]{217} \sin{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3}{2} + i \left(- \sqrt[4]{217} \cos{\left(\frac{\operatorname{atan}{\left(17 \sqrt{3} \right)}}{2} \right)} - \frac{3 \sqrt{3}}{2}\right)\right)$$

$$512$$

Simplify

Numerical answer [src]

x1 = 1.16748194582983 + 0.161536067813473*i

x2 = 1.16748194582983 - 0.161536067813473*i

x3 = -4.16748194582983 + 5.3576884905201*i

x4 = -4.16748194582983 - 5.3576884905201*i

x5 = 2.0

x6 = 4.0

x6 = 4.0

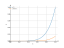
The graph

Other calculators

How to use it?

Identical expressions

Similar expressions

x^6=(6x-8)^3 equation

Numerical solution:

The solution